This item is taken from IGCSE Mathematics (0580) Paper 4 of October/November 2014.
To deal with this item, let us summarize the details of the problem:
(a) Syllabus area: Numbers / Algebra
(b) Specific topic: Limits of Accuracy
(c) Formula Needed:
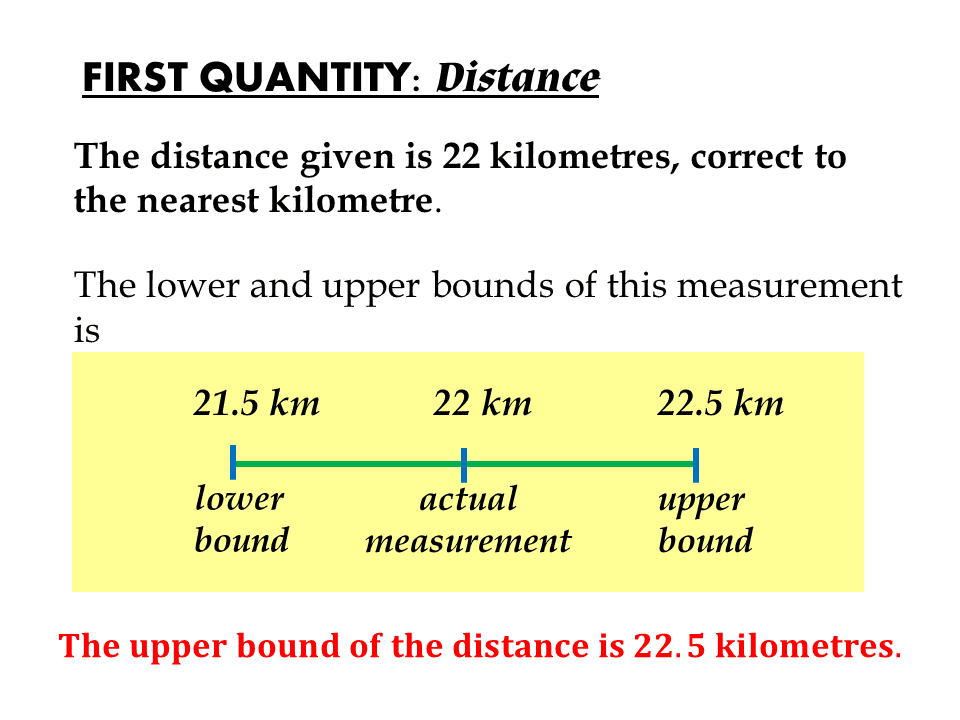
Let us start with the first quantity, which is the distance. We should take note that the distance is given correct to the nearest kilometre. In other words, it is correct to the nearest whole number. It is then expected that the bounds would consist of one (1)
decimal place, which round off to the whole number.
The second quantity is the time. We take note that it is given correct to the nearest half hour. To get the lower and upper bounds of the time, let us first illustrate how to round off to the nearest half hour, given the 2.5 in the middle.
We should take note that 2 to 2.5 is half an hour; the same case for 2.5 to 3. If we look at the number line, starting from 2, before it round off to the nearest half hour, it should reach first halfway between 2 and 2.5. It means that if it reaches 2.25, it can be rounded off to 2.5 already. The same case for the other side. At 2.75, it will round off to 3 already. These numbers then are the lower and upper bounds of 2.5.
The upper bound then for the speed will be
Therefore, the upper bound of the speed is 10 km/h.
---------------------------------------------------------------------------------------------------------------
Your comments and suggestions are welcome here. Write them in the comment box below.
Thank you and God bless!
To deal with this item, let us summarize the details of the problem:
(a) Syllabus area: Numbers / Algebra
(b) Specific topic: Limits of Accuracy
(c) Formula Needed:
Let us start with the first quantity, which is the distance. We should take note that the distance is given correct to the nearest kilometre. In other words, it is correct to the nearest whole number. It is then expected that the bounds would consist of one (1)
decimal place, which round off to the whole number.
The second quantity is the time. We take note that it is given correct to the nearest half hour. To get the lower and upper bounds of the time, let us first illustrate how to round off to the nearest half hour, given the 2.5 in the middle.
We should take note that 2 to 2.5 is half an hour; the same case for 2.5 to 3. If we look at the number line, starting from 2, before it round off to the nearest half hour, it should reach first halfway between 2 and 2.5. It means that if it reaches 2.25, it can be rounded off to 2.5 already. The same case for the other side. At 2.75, it will round off to 3 already. These numbers then are the lower and upper bounds of 2.5.
The upper bound then for the speed will be
Therefore, the upper bound of the speed is 10 km/h.
---------------------------------------------------------------------------------------------------------------
Your comments and suggestions are welcome here. Write them in the comment box below.
Thank you and God bless!

%2BProblem.PNG)






















No comments:
Post a Comment
Write your comments and suggestions here.